このページでは、それが何であるか、そして 2 つのベクトルの内積を計算する方法を説明します。また、内積を使用して 2 つのベクトル間の角度を見つける方法と、さらに内積のすべてのプロパティも学習します。最後に、段階的に解決された例と演習を使って練習できるようになります。
2 つのベクトル間の内積を計算する方法
数学におけるドット積は、2 つのベクトルを乗算して実数に変換するベクトル演算です。したがって、2 つのベクトルの内積を計算するには 2 つの方法があります。
2 つのベクトルの座標がわかっている場合は、X 成分と Y 成分を掛け合わせて結果を加算することで、それらの内積を求めることができます。言い換えると、2 つのベクトルがあるとします。
![]()
それらの間の内積は次のとおりです。
![]()
たとえば、次の 2 つのベクトルの内積は次のようになります。
![]()
![Rendered by QuickLaTeX.com \displaystyle \begin{aligned} \vv{\text{u}} \cdot \vv{\text{v}}&=(1,2)\cdot (-1,3) \\[1.5ex]&=1\cdot (-1) + 2 \cdot 3 \\[1.5ex] & = -1+6 \\[1.5ex] & =\bm{5} \end{aligned}](https://mathority.org/wp-content/ql-cache/quicklatex.com-756e86b9b5b7a9f5df2cacf728d82855_l3.png)
これは、2 つのベクトル間の内積を見つける方法です。ただし、別の方法もあります。
一方、モジュールと 2 つのベクトル間の角度がわかっている場合は、モジュールの積とそれらが形成する角度の余弦を計算することで、2 つのベクトル間のスカラー積を決定できます。
![]()
金
![]()
そして
![]()
ベクトルのモジュールです
![]()
そして
![]()
それぞれと
![]()
彼らが作る角度。
ベクトルの大きさはその成分の二乗根であることを思い出してください。
![]()
例として、モジュールとそれらの間の角度が次のような 2 つのベクトルのスカラー積を解きます。
![]()
![Rendered by QuickLaTeX.com \displaystyle \begin{aligned} \vv{\text{u}} \cdot \vv{\text{v}} & = \lvert \vv{\text{u}} \rvert \cdot \lvert \vv{\text{v}} \rvert \cdot \cos(\alpha ) \\[1.5ex] &= 3 \cdot 4 \cdot \cos(60º)\\[1.5ex] & = 3 \cdot 4 \cdot 0,5 \\[1.5ex] &= \bm{6} \end{aligned}](https://mathority.org/wp-content/ql-cache/quicklatex.com-a363625d93f01339437d5ad065050025_l3.png)
一方、ドット積は、ドット積、スカラー積、ドット積とも呼ばれます。
注:内積と外積を混同しないでください。名前は似ていますが、概念はまったく異なります。
内積を使用して 2 つのベクトルの間の角度を見つけます
内積の定義を見ると、2 つのベクトルを乗算する目的は何なのか疑問に思うかもしれません。そうですね、内積の応用の 1 つは、2 つのベクトルによって形成される角度を計算することです。

内積公式の余弦を解くと、次が得られます。
![]()
例を通してこれがどのように行われるかを見てみましょう。
- 次の 2 つのベクトル間の角度を求めます。
![]()
まず、2 つのベクトルの大きさを見つける必要があります。
![]()
![]()
次に、次の式を使用して 2 つのベクトル間の角度の余弦を計算します。
![]()
最後に、電卓を使用してコサインの逆関数を実行して、対応する角度を見つけます。
![]()
したがって、ベクトルは 74.93 度の角度を形成します。
2 つのベクトルの内積のプロパティ
内積には次の特性があります。
- 可換性: ベクトルを乗算する順序は関係ありません。
![]()
- 分配的性質: ドット積はベクトルの加算と減算に関して分配的です。
![]()
![]()
- 連想プロパティ: 結果が等しいため、演算の実行前または実行後にドット積に定数を乗算できます。
![]()
- 2 つのベクトルが直交(または直交) している場合、それらの内積は 0 になります。 2 つの直交するベクトルの角度は 90 度であり、90 度のコサインは 0 に等しいため、この特性は簡単に実証できます。
![Rendered by QuickLaTeX.com \displaystyle \begin{aligned} \vv{\text{u}} \cdot \vv{\text{v}} & = \lvert \vv{\text{u}} \rvert \cdot \lvert \vv{\text{v}} \rvert \cdot \cos(90º ) \\[1.5ex] &=\lvert \vv{\text{u}} \rvert \cdot \lvert \vv{\text{v}} \rvert \cdot 0 \\[1.5ex] &= 0 \end{aligned}](https://mathority.org/wp-content/ql-cache/quicklatex.com-77e3b216b12f49a9dde99c0fbf626658_l3.png)
- 逆に、2 つのベクトルが平行である場合、それらのスカラー積はモジュールの積と同じになります。同じ方向の 2 つのベクトルが 0° の角度を形成し、そのコサインが 1 に等しいため、この特性も簡単に検証できます。
![Rendered by QuickLaTeX.com \displaystyle \begin{aligned} \vv{\text{u}} \cdot \vv{\text{v}} & = \lvert \vv{\text{u}} \rvert \cdot \lvert \vv{\text{v}} \rvert \cdot \cos(0º) \\[1.5ex] &=\lvert \vv{\text{u}} \rvert \cdot \lvert \vv{\text{v}} \rvert \cdot 1 \\[1.5ex] &= \lvert \vv{\text{u}} \rvert \cdot \lvert \vv{\text{v}} \rvert \end{aligned}](https://mathority.org/wp-content/ql-cache/quicklatex.com-777ec2544cac64aa065df21872989b4a_l3.png)
- 最後に、ベクトル自体の内積は、その大きさの 2 乗に相当します。
![]()
2 つのベクトル間のスカラー積問題を解決しました
演習 1
次の 2 つのベクトルの平面内の内積を計算します。
![]()
2 つのベクトルの内積を計算するには、X 座標と Y 座標を掛け合わせて、結果を加算する必要があります。
![Rendered by QuickLaTeX.com \displaystyle \begin{aligned}\vv{\text{u}} \cdot \vv{\text{v}} & = (4,-3)\cdot (5,2) \\[1.5ex] & = 4\cdot 5 + (-3) \cdot 2 \\[1.5ex] & = 20-6\\[1.5ex] & =\bm{14} \end{aligned}](https://mathority.org/wp-content/ql-cache/quicklatex.com-f9cb0f372eee67ad149d5b2cff8d2f99_l3.png)
演習 2
モジュールとそれらが形成する角度が次のような 2 つのベクトルのスカラー積を求めます。
![]()
それらのモジュールとそれらの間の角度がわかっているので、内積公式を直接適用できます。
![Rendered by QuickLaTeX.com \displaystyle \begin{aligned} \vv{\text{u}} \cdot \vv{\text{v}} & = \lvert \vv{\text{u}} \rvert \cdot \lvert \vv{\text{v}} \rvert \cdot \cos(\alpha ) \\[1.5ex] &= 6 \cdot 3 \cdot \cos(45º)\\[1.5ex] & = 6 \cdot 3 \cdot 0,71 \\[1.5ex] &= \bm{12,73} \end{aligned}](https://mathority.org/wp-content/ql-cache/quicklatex.com-38999386ccbd92758c9968f025beff72_l3.png)
演習 3
次の 2 つのベクトル間の角度はいくらですか?
![]()
まず、2 つのベクトルの大きさを計算する必要があります。
![]()
![]()
次の式を使用して、ベクトルによって形成される角度の余弦を計算します。
![]()
そして最後に、計算機を使用してコサインの逆関数を実行して、対応する角度を見つけます。
![]()
演習 4
次の 2 つのベクトルを考えてみましょう。
![]()
次の演算を計算します。
![]()
まず括弧内のドット積を解く必要があり、次に括弧の外側のドット積による乗算を行う必要があります。
![]()
![]()
![]()
![]()
![]()
![]()
演習 5
次の 3 つの 2 次元ベクトルがあるとします。
![]()
次の演算を計算します。
![]()
まず、ベクトルに括弧内のスカラーを乗算します。
![]()
![]()
![]()
ここでベクトルの減算を行います。
![]()
![]()
そして最後に、スカラー積を解きます。
![]()
![]()
![]()
演習 6
の値を計算します
![]()
次のベクトルが垂直になるようにします。
![]()
2 つの直交するベクトルは 90 度の角度を形成します。したがって、cos(90°)=0 であるため、角度の余弦はゼロでなければなりません。まだ:
![]()
![]()
分数の分母は方程式の右側全体を除算するので、もう一方の側で乗算することでそれを渡すことができます。
![]()
![]()
ここでスカラー積を解きます。
![]()
![]()
![]()
そして最後に、未知の部分を明らかにします。
![]()
![]()
![]()
演習 7
角度を計算する
![]()
そして
![]()
これらは次の三角形の辺を形成します。
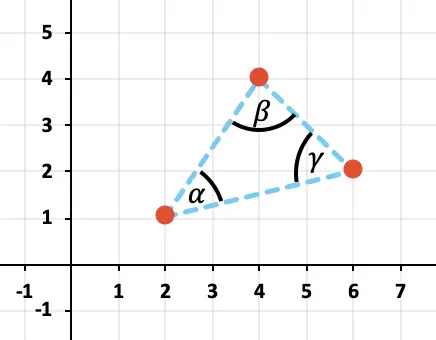
三角形を構成する頂点は次の点です。
![]()
三角形の内角を計算するには、その各辺のベクトルを計算し、内積公式を使用してそれらが形成する角度を見つけます。
たとえば角度を求めるには
![]()
その辺のベクトルを計算します。
![]()
![]()
そして、内積公式を使用して 2 つのベクトルによって形成される角度を求めます。
![]()
![]()
![]()
![]()
同じ手順を繰り返して角度を決定します
![]()
![]()
![]()
![]()
![]()
最後に、最後の角度を見つけるために、同じ手順を繰り返すことができます。ただし、三角形のすべての角度の合計は 180 度になる必要があるため、次のようになります。
![]()